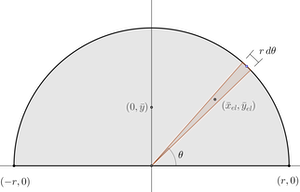
First, watch this Khan Academy Video where Sal discusses how to integrate using polar coordinates. In particular, he shows that the differential element of area in polar coordinates is \(dA = \frac{1}{2} r^2\, d\theta \). Then prove, using integration with polar coordinates, that the area of a semi-circle is $\pi r^2$ and that $\bar{y} = \frac{4 r}{3 \pi}$.
Hints:
- The equation of a circle with radius $r$ in polar coordinates is $r(\theta) = r$.
- The process to find area and centroids is exactly the same as previous problems, only the form of the integrals is different.
- Finding the area of a circle using polar coordinates is trivially easy.
- To find $Q_x$ you will need to determine $\bar{y}_{el}$ as a function of $r$ and $\theta$.